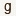Exercise 2.7

---

1. Consider a concept learning problem in which each instance is a real number, and in which each hypothesis is an interval over the reals. More precisely, each hypothesis in the hypothesis space H is of the form a < x < b, where a and b are any real constants, and x refers to the instance. For example, the hypothesis 4.5 < x < 6.1 classifies instances between 4.5 and 6.1 as positive, and others as negative. Explain informally why there cannot be a maximally specific consistent hypothesis for any set of positive training examples.
   
   There cannot be because there always exists another degree of precision, as in another digit in the decimal portion of the real number.
   
   
2. Suggest a slight modification to the hypothesis representation so that there will be.
   
   There could be a limit set on the number of decimal places on a valid real representation in the hypothesis language.
   
   Note: I could use <= instead.

---